Matematikë, Gjimnazi Natyror A 2012
Pyetja 1
Sa është vlera e
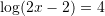 ?
?A.
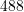
B.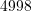
C.
D.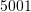
Zgjidhje:


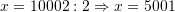
Pyetja 2
Në qoftë se vlenë
 , sa është vlera e
, sa është vlera e  ?
?A. – 2
B. 2
C. -7
D. 8Zgjidhje:

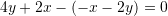
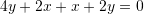
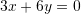
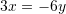
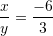

Pyetja 3
Është dhënë funksioni
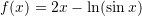 . Sa është
. Sa është 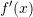 ?
?A.

B.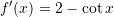
C.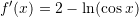
D.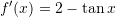
Zgjidhje:
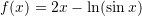
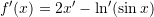
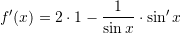
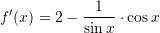
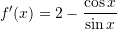
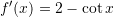
Pyetja 4
Nëse
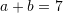 dhe
dhe 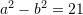 sa është vlera e
sa është vlera e 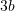 ?
?A.

B.
C.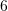
D.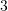
Zgjidhje:
Nga
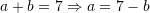
Kemi,
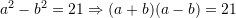
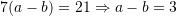
Zëvendësojmë
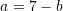
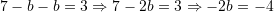
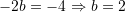
Atëherë
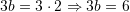
Pyetja 5
Tabela vijuese tregon vlerat e një funksioni
 dhe derivatit të tij
dhe derivatit të tij  .
.
x f (x) f ‘(x)
0 2 5
1 4 -3
2 -2 3
3 4 2Nëse funksioni
 është dhënë me
është dhënë me  , caktoni
, caktoni  .
.A.
B.
C.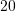
D.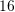
Zgjidhje:
Nga
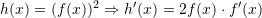
Në rastin tonë
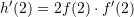
Nga tabela
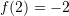 dhe
dhe 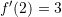
Atëherë
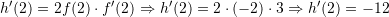
Pyetja 6
Janë dhënë barazimet
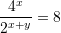 dhe
dhe 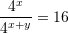 . Sa është vlera e
. Sa është vlera e  ?
?A. – 2
B. – 4
C. 2
D. 4Zgjidhje:
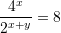
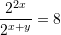
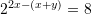
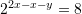

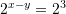
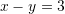
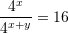
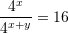

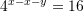
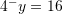
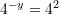

Gjejmë
 -in nga
-in nga 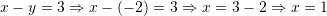
Kështu
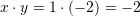
Pyetja 7
Nëse
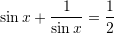 sa është vlera e shprehjes
sa është vlera e shprehjes 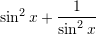
A.

B.
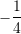
C.
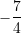
D.

Zgjidhje:
Pyetja 8
Cili është forma kanonike e ekuacionit të hiperbolës nëse
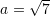 dhe
dhe 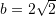 ?
?A.
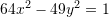
B.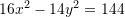
C.
D.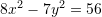
Zgjidhje:
Ekuacioni i hiperbolës në formë kanonike është:
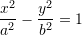
Në rastin tonë, për
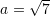 dhe
dhe 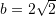 , ekuacioni merr formën
, ekuacioni merr formën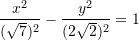
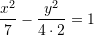
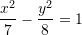
Shumëzojmë ekuacionin me numrin
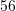
Kemi,
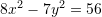
Pra, forma kanonike e ekuacionit të hiperbolës është
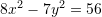
Pyetja 9
Në trekëndëshin ABC, janë dhënë
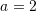 ,
, 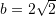 dhe
dhe 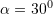 . Zbato teoremen e sinusit dhe trego se cila është vlera e këndit
. Zbato teoremen e sinusit dhe trego se cila është vlera e këndit 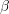 ?
?A.
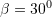
B.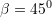
C.
D.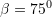
Zgjidhje:
Teorema e Sinusit thotë:
“Në cdo trekëndësh brinjët janë proporcionale me sinuset e këndeve përballë tyre; herësi është i barabartë me diametrin e rrethit të jashtëshkruar në atë trekëndësh.”
Pra,
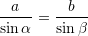
Prej nga,
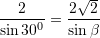
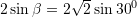
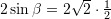
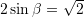
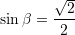

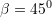
Pyetja 10
Është dhënë funksioni
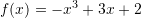 . Cili nga pohimet është i saktë ?
. Cili nga pohimet është i saktë ?A. Funksioni është tek.
B. Funksioni ka asimptotë horizontale.
C. Funksioni ka shkëputje në pikën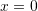 .
.
D. Funksioni ka vlera ekstreme për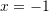 dhe
dhe 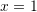










pse nuk jan pergjigjjet e grupit B
sepse ardit nuk kemi mundur ti gjejme keshtu nese keni ndonje daj ne zvicerr thuj mu mi ble 1 par patika adidas se kom met veq npapuqe mpuq nga bibi